Minor Scales

Three Types of Minor Scales – Oh My!

scale-a harmonic-half The scales that represent the three minors present one of the biggest challenges for music students.

This section will review and clarify the application and use of these scales.

The three types of minors present a dilemma as to what each is meant to accomplish.

The in depth reasons of the whys and whats can be accomplished through research. The foundation of the how is what is covered here.

An exploration of the math and simple explanations for the beginning student are provided to familiarize the student in recognizing the minor types of scale and the potential use or sound that may be applied.

Your understanding of scale theory will provide a basis for creative composition, harmonizing and improvisation.

The Minor Scales The continuation of a eight note scale applies to minor scales. The key for these scales is to flat various intervals which provide a new sound. Each variation has its own unique sound and emotion.

There is a very specific math formula to based on the flat intervals for each type of scale. The starting note is the root note and is the name of the Key signature and minor scale. It varies in what it is called by the flats applied. Let’s take a look.

The Three Scale Types for Minors

Natural
Harmonic
Melodic

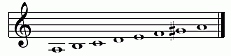
The following three examples of a the scale for A minor

A Major scale has a C#, F#, and G#. the natural minor will flat these three notes.

Natural Minor a.k.a relative minor (same notes as C major)
3 flats – 3rd, 6th, & 7th

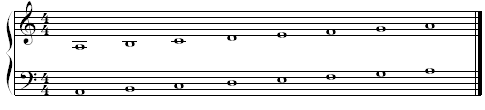
Harmonic Minor
2 flats – 3rd & 6th

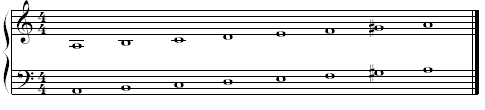
Melodic Minor
1 flat – 3rd

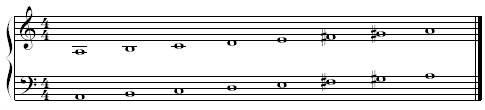
One of the first things to notice is the number of flat notes and how the first natural minor has 3 flats and then each change in minor scale drops off a flat key. This is a great pattern to recognize right off the start.

Major and Minor Key and Scale Relationships

Below we will compared the C Key signature and the various minor scales produced to the same C start point. That is done to help you see everything in a one key related. However, we have to start with the what the minor keys are related to first.

The minor scale is related to the major scale by a 6th interval up or a minor 3rd interval down. For the key of C minor the related major is E major.

Compare Eb major with the C natural minor scale – they use the same notes. Only the starting positions change.

Eb Major

Eb + F + G + Ab + Bb + C + D + Eb
C – D – Eb + F + G + Ab + Bb + C
C Natural Minor

From here the other variations are used for harmonic and melodic minors. This is the practical knowledge you need to know about minors.

In addition we can modify several intervals to ‘flat’ and call them minor intervals. The natural minor with three flat intervals will be as follows for the C minor scale.
Intervals

1 – 2 – m3 – 4 – 5 – m6 – m7 – 8

C – D – Eb – F – G – Ab – Bb – C

Constructing Minors – The Scale Math

We will use the S = Start position then add the steps to construct a scale.

Start with remembering the Major Scale for C with intervals, steps, and notes.

1 - 2 - 3 - 4 - 5 - 6 - 7 - 8 
 
 S + W + W + H + W + W + H + W   
 

C + D + E + F + G + A + B + C

 on the keyboard:

Then compare the minor scales. Normally we would start with a natural minor related to the major scale a 3rd down. In this case we are going to go ahead and complete them in the same key to see the math differences.

Mathematically the natural minor is produced using the following formula for making the 3rd, 6th, and 7th intervals minor.

1 + 2 + m3 + 4 + 5 + m6 + m7 + 8
 
  S + W + H  + W + W + H  + W  + W  
 

C + D + Eb + F + G + Ab + Bb + C

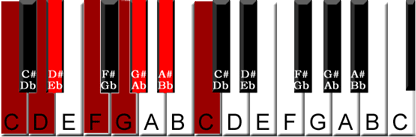
Mathematically the harmonic minor is produced using the following formula or making the 3rd and 6th intervals minor.

1 + 2 + m3 + 4 + 5 + m6 + 7 + 8
 
   S + W + H  + W + W + W + 3H + H   
 

C + D + Eb + F + G + Ab + B + C

notice the 3 half steps from m6 to 7.

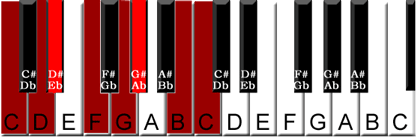
Mathematically the melodic minor is produced using the following formula for only the 3rd as minor.

1 + 2 + m3 + 4 + 5 + 6 + 7 + 8
 
   S + W + H  + W + W + W + W + H   
 

C + D + Eb + F + G + A + B + C

notice the 4 whole steps in a row.

You can create all the minor scales using these formulas. By applying additional music theory as learned in the key signature section you can build scales and see relationships between the various keys signatures and scale building.

Using Scales that are Minor

Natural Minors are an easy extension of the major scale and can be applied in the major key signatures because they use the same notes.

Harmonic Minors are used for many of the classical works. They have a sad or melancholy sound to them.

Melodic Minors are used extensively in jazz improvisation. In classical theory the melodic minor is played going up the scale, but when coming down the natural minor is used. With modern jazz the melodic minor scale is used going up and down.

What is probably most important is that the members of band all know which scale you are using. In advanced theory many types of scales can be used so it becomes even more important.

Learning Resources

Scales Workshop

Learning scales is intimidating for the beginning student.